Question

A manufacturer receives parts from two suppliers. A simple random sample of 400 parts from supplier 1 finds 20 defective. A simple random sample of 200 parts from supplier 2 finds 20 defective. Let p1 and p2 be the proportion of all parts from suppliers 1 and 2, respectively, that are defective. Test whether the defective rates of the parts from two suppliers are significant different at the 1% significance level. Conduct a hypothesis testing. Answer the next three questions. 12. Test statistic

Answer 1

The test statistic is
`z = -2.1`

The p-value of the test is 0.0358 > 0.01, which means that the defective rates of the two suppliers are not significant different at the 1% significance level.

Explanation:

Before testing the hypothesis, we need to understand the central limit theorem and subtraction of normal variables.

Central Limit Theorem

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

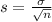For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean
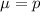`\mu = p` and standard deviation
`s = \sqrt{(p(1-p))/(n)}`

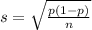
Subtraction between normal variables:

When two normal variables are subtracted, the mean is the difference of the means, while the standard deviation is the square root of the sum of the variances.

A simple random sample of 400 parts from supplier 1 finds 20 defective.

This means that:

`p_1 = (20)/(400) = 0.05, s_1 = \sqrt{(0.05*0.95)/(400)} = 0.0109`

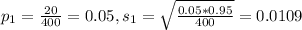
A simple random sample of 200 parts from supplier 2 finds 20 defective.

This means that:

`p_2 = (20)/(200) = 0.1, s_2 = \sqrt{(0.1*0.9)/(200)} = 0.0212`

Let p1 and p2 be the proportion of all parts from suppliers 1 and 2, respectively, that are defective. Test whether the defective rates of the parts from two suppliers are significant different at the 1% significance level.

At the null hypothesis, we test if they are equal, that is, if the subtraction of the proportions is 0. So

`H_0: p_1 - p_2 = 0`

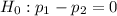
At the alternate of the null hypothesis, we test if they are different, that is, if the subtraction of the proportions is different of 0. So

`H_a: p_1 - p_2 \\eq 0`

The test statistic is:

`z = (X - \mu)/(s)`

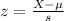
In which X is the sample mean,
`\mu` is the value tested at the null hypothesis and s is the standard error.

0 is tested at the null hypothesis:

This means that
`\mu = 0`

From the sample proportions:

`X = p_1 - p_2 = 0.05 - 0.1 = -0.05`

`s = √(s_1^2+s_2^2) = √(0.0109^2 + 0.0212^2) = 0.0238`

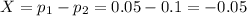
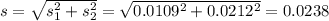
Value of the test statistic:

`z = (X - \mu)/(s)`

`z = (-0.05 - 0)/(0.0238)`

`z = -2.1`

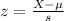
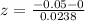
P-value of the test and decision:

The p-value of the test is the probability that the sample proportion differs from 0 by at least 0.05, that is, P(|z| < 2.1), which is 2 multiplied by the p-value of Z = -2.1.

Z = -2.1 has a p-value of 0.0179

2*0.0179 = 0.0358.

The p-value of the test is 0.0358 > 0.01, which means that the defective rates of the two suppliers are not significant different at the 1% significance level.