Question

How much fencing is required to enclose a circular garden whose radius is 14​m? Use 22/7 for π.

Answer 1

The fencing required to enclose a circular garden whose radius is 22m is 138.16m

Answer 2

`\boxed {\boxed {\sf 88 \ meters}}`

Explanation:

We want to find the amount of fencing required to enclose the garden. The fencing goes around the garden, which is a circle. Essentially, we are finding the perimeter or circumference of the circle.

The formula for circumference is:

`c=\pi * d`

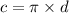
We are given the radius, so we need to find the diameter. The radius goes from the center to the edge of the circle, while the diameter goes from edge to edge through the center. So, the diameter is twice the radius of 14 meters.

• d=2 ×r
• d= 2 × 14 m
• d= 28 m

Now we know the diameter is 28 meters. We also know 22/7 is being used for pi. Substitute these values into the formula.

`c= (22)/(7) * 28 \ m`

`c=88 \ m`

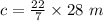
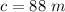
The circumference is 88 meters, so 88 meters of fencing is requires to enclose the garden.