1 Answer

Martin Booth · Answer 1 · 2022-02-25T12:44:25+0000

Given:

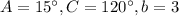$A=15^\circ, C=120^\circ,b=3$ .

To find:

The length of side c.

Solution:

According to the angle sum of property of a triangle, the sum of all interior angles of a triangle is 180 degrees.

$m\angle A+m\angle B+m\angle C=180^\circ$

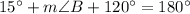
$15^\circ+m\angle B+120^\circ=180^\circ$

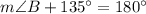
$m\angle B+135^\circ=180^\circ$

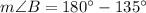
$m\angle B=180^\circ-135^\circ$

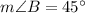
$m\angle B=45^\circ$

According to the Law of sines,

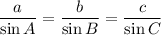
$(a)/(\sin A)=(b)/(\sin B)=(c)/(\sin C)$

Using Law of sines, we get

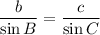
$(b)/(\sin B)=(c)/(\sin C)$

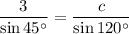
$(3)/(\sin 45^\circ)=(c)/(\sin 120^\circ)$

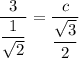
(3)/((1)/(√(2)))=(c)/((√(3))/(2))

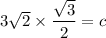
3√(2)* (√(3))/(2)=c

On further simplification, we get

(3√(6))/(2)=c

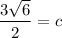
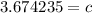
3.674235=c

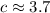
$c\approx 3.7$

Therefore, the length of side c is about 3.7 units.

Please log in or register to add a comment.