Answer:
The test statistic is

Explanation:
First, before finding the test statistic, we need to understand the central limit theorem and difference between normal variables.
Central Limit Theorem
The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
 and standard deviation
and standard deviation
 , the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
 and standard deviation
and standard deviation
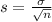 .
.
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean
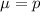 and standard deviation
and standard deviation
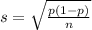
Subtraction between normal variables:
When two normal variables are subtracted, the mean is the difference of the means, while the standard deviation is the square root of the sum of the variances.
A random sample of children in two different schools found that 16 of 40 at one school
This means that:

13 of 30 at the other had this infection.
This means that:
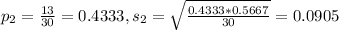
Conduct a test to answer if there is sufficient evidence to conclude that a difference exists between the proportion of students who have ear infections at one school and the other.
At the null hypothesis, we test if there is no difference, that is:
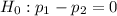
And at the alternate hypothesis, we test if there is difference, that is:

The test statistic is:
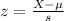
In which X is the sample mean,
 is the value tested at the null hypothesis and s is the standard error
is the value tested at the null hypothesis and s is the standard error
0 is tested at the null hypothesis:
This means that

From the two samples:

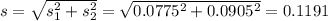
Value of the test statistic:
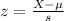
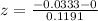

The test statistic is
