Question

The radius of a sphere is increasing at a rate of 5 mm/s. How fast is the volume increasing (in mm3/s) when the diameter is 60 mm

Answer 1

The volume of the sphere is increasing at a rate of 56,549 cubic millimeters per second.

Explanation:

Volume of a sphere:

The volume of a sphere is given by:

`V = (4\pi r^3)/(3)`

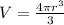
In which r is the radius.

Solving this question:

The first step do solve this question is derivating V implictly in function of t. So

`(dV)/(dt) = 4\pi r^2 (dr)/(dt)`

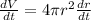
The radius of a sphere is increasing at a rate of 5 mm/s.

This means that
`(dr)/(dt) = 5`

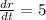
Diameter is 60 mm

This means that
`r = (60)/(2) = 30`

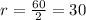
How fast is the volume increasing (in mm3/s) when the diameter is 60 mm?

This is
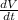`(dV)/(dt)`. So

`(dV)/(dt) = 4\pi r^2 (dr)/(dt)`

`(dV)/(dt) = 4\pi*30^2*5 = 900*20\pi = 56549`

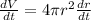
The volume of the sphere is increasing at a rate of 56,549 cubic millimeters per second.