Question

At age 23​, someone sets up an IRA​ (individual retirement​ account) with an APR of ​7%. At the end of each month he deposits ​$75 in the account. How much will the IRA contain when he retires at age​ 65? Compare that amount to the total deposits made over the time period.

Answer 1

The ira will contain $228,278.05 when he retires at age 65. This is 6.04 times the amount of money he deposited.

Explanation:

In order to solve this problem, we can make use of the following formula:

`FV=PMT[((1+i)^(n)-1)/(i)]`

Where:

FV= Future value of the ira

PMT= the amount of money you deposit each month

i= is the interest rate per period

n=number of periods

in this case we will assume the interest will be compounded each month.

So:

FV this is what we need to know.

PMT= $75 the amount he will deposit each month

t = 42 years,

this is 65-23=42

n=42 years * 12 months/year = 504 months

i=0.07/12

So we can now use the given formula:

`FV=PMT[((1+i)^(n)-1)/(i)]`

`FV=75[((1+(0.07)/(12))^(504)-1)/((0.07)/(12))]`

So we get:

FV=$228,278.05

which is the amount of money he will have after 42 years.

In total, he deposited:

$75*504months = $37,800

so he will have:

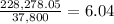`(228,278.05)/(37,800)=6.04` times the amount of money he deposited throughout this time.