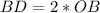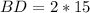Question

In rhombus ABCD shown below, AB=17 and AC=16 determine the length of BD without (hint , not with trigonometry)

Answer 1

`BD = 30`

Explanation:

Given

`AB = 17`

`AC = 16`

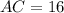
See attachment

Required

Find BD

If AC = 16, then:

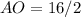`AO = 16/2` i.e. half the diagonal AC

`AO = 8`

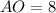
The diagonals of a rhombus are perpendicular.

This implies that we can apply Pythagoras theorem.

Using Pythagoras theorem on triangle AOB, we have:

`AB^2 = AO^2 + OB^2`

`17^2 = 8^2 + OB^2`

`289 = 64 + OB^2`

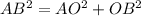
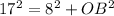
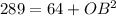
Collect like terms

`OB^2 = 289 - 64`

`OB^2 = 225`

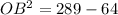
Take positive square roots of both sides

`OB = 15`

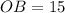
To solve for BD, we use:

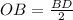`OB = (BD)/(2)` --- i.e. half the diagonal BD

`BD = 2 * OB`

`BD = 2 * 15`

`BD = 30`