Solution :
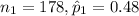
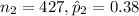
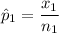
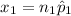
= 178 x 0.48
= 85.44
≈ 85
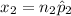
= 427 x 0.38
= 162.26
≈ 162
a). Yes, the sample sizes are large enough to use the large sample confidence interval so as to estimate the difference in the population proportions.
Let

Where the
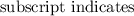 the age
the age
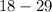 group and the 2 - subscript indicates the age
group and the 2 - subscript indicates the age
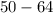 group.
group.
Since

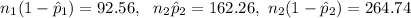
are all at least 10, the sample sizes are large enough to use the large sample confidence interval.
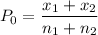
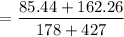
= 0.409421

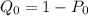
= 1 - 0.4094
= 0.5906
b). 90% confidence interval is
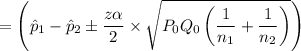
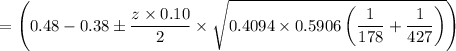
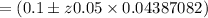

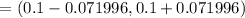
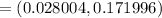
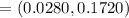
c). Zero is not included in the confidence interval. Answer is no. The difference in the two population proportion are different from each other.