Question

A beacon is flashing on top of a 50 foot tower. A 6 foot tall man walks constantly away from the tower at 5 feet/sec. At the instant the man is 30 feet away from the tower, what rate is the tip of his shadow moving away from the tower

Answer 1

Answer:
`(253)/(44)`

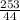
Explanation:

ignore the "at the instant the man is 30 feet away" part, set it as X and the man's shadow as Y.

Similar triangles so we can do
`(50)/(x+y) = (6)/(y)`.

Solve for it we get 44y = 6x

Differentiate relative to time t, we get 44y' = 6x'.

change in x (x') is equal to 5. And we get the answer y' =
`(33)/(44)`.

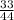the
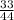`(33)/(44)` ft/sec is the rate of which the length of the shadow is changing. add 5 to it for the rate of the tip of his shadow moving away from the tower.