Answer:
The ball will be at a height of 3 feet above the ground after 3.729 seconds.
Explanation:
Let suppose that the tennis player has hit the ball vertically, meaning that ball will experiment a free fall, that is, an uniform accelerated motion due to gravity. The time taken by the ball in terms of its initial velocity (
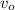 ), in feet per second, initial position (
), in feet per second, initial position (
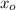 ), in feet, final position (
), in feet, final position (
 ), in feet, and gravitational acceleration (
), in feet, and gravitational acceleration (
 ), in feet per square second is described by this second order polynomial:
), in feet per square second is described by this second order polynomial:
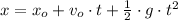 (1)
(1)
If we know that
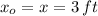 ,
,
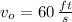 and
and
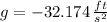 , then the time needed by the ball to be at a height of 3 feet again is:
, then the time needed by the ball to be at a height of 3 feet again is:
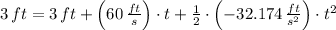
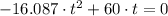 (2)
(2)
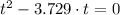
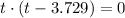
The binomial contains the time when the ball will be at a height of 3 feet again. In other words, the ball will be at a height of 3 feet above the ground after 3.729 seconds.