In similar triangles ABC and ADE, the true statements are: B.
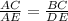 , D.
, D.
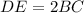 , E.
, E.
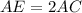 .
.
The given answer choices involve properties of similar triangles ABC and ADE. Statements B, D, and E are true for similar triangles:
B.
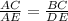 is true by the definition of similar triangles.
is true by the definition of similar triangles.
D.
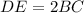 is true as corresponding sides of similar triangles are in proportion.
is true as corresponding sides of similar triangles are in proportion.
E.
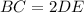 is false, as it's the reverse of statement D.
is false, as it's the reverse of statement D.
Statement F is incorrect, as AC cannot be equal to
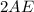 in similar triangles.
in similar triangles.
Therefore, B, D, and E must be true for the given pair of similar triangles.