Question

What is the equation in slope-intercept form of the line that crosses the x-axis at 21 and is perpendicular to the line represented by y = 7/2x-13

Answer 1

Given:

A line is perpendicular to the line
`y=(7)/(2)x-13`.

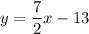The line passes through the x-axis at 21.

To find:

The equation of the line in slope intercept form.

Solution:

The slope intercept form of a line is:

`y=mx+b`

Where, m is the slope and b is the y-intercept.

The given line is:

`y=(7)/(2)x-13`

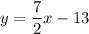
So, the slope of this line is
`(7)/(2)`.

The product of slopes of two perpendicular lines is -1.

Let m be the slope of required line. Then the slope of the required line is:

`m* (7)/(2)=-1`

`m=-(2)/(7)`

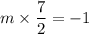
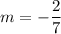
The line passes through the x-axis at 21. It means the line passes through the point (21,0). So, the equation of the line is:

`y-y_1=m(x-x_1)`

`y-0=-(2)/(7)(x-21)`

`y=-(2)/(7)(x)-(2)/(7)(-21)`

`y=-(2)/(7)x+6`

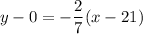
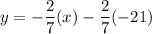
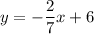
Therefore, the equation of the required line is
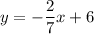`y=-(2)/(7)x+6`.