Question

The following estimated regression equation was developed for a model involving two independent variables.

ŷ​ =40.7+8.63x1​+2.71x^2​

After x2 was dropped from the model, the least squares method was used to obtain an estimated regression equation involving only x1 as an independent variable.

ŷ= 42.0+9.01x1
​
Required:
a. Give an interpretation of the coefficient of x1 in both models.
b. Could multicollinearity explain why the coefficient of x1 differs in the two models? If so, how?

Answer 1

(a): y increases on average by 8.63/unit of x1 in the first equation and increases on average by 9.01/unit of x1 in the second

(b): Yes

Explanation:

Given

`\wedge y = 40.7 + 8.63x_1 +2.71x_1`

`\wedge y = 42.0 + 9.01x_1`

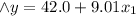
Solving (a): An interpretation of x1 coefficient

We have the coefficients of x1 to be 8.63 and 9.01

Literally, the coefficient represents the average change of y-variable per unit increase of the dependent variable

Since the coefficients of x1 in both equations are positive, then that represents an increment on the y variable.

So, the interpretation is:

y increases on average by 8.63/unit of x1 in the first equation and increases on average by 9.01/unit of x1 in the second

Solving (b): Multicollinearity

This could be the cause because x1 and x2 are related and as a result, x2 could take a part of the coefficient of x2