Given:
f(x) and g(x) are two quadratic functions.

The table of values for the function g(x) is given.
To find:
The statement that best compares the maximum value of the two functions.
Solution:
We have,

Here, the leading coefficient is -8 which is a negative number. So, the function f(x) represents a downward parabola.
We know that the vertex of a downward parabola is the point of maxima.
The vertex of a quadratic function
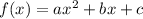 is:
is:

In the given function,
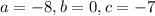 .
.
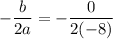
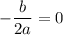
Putting
 in the given function, we get
in the given function, we get
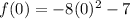
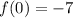
So, the vertex of the function f(x) is at (0,-7). It means the maximum value of the function f(x) is -7.
From the table of g(x) it is clear that the maximum value of the function g(x) is 6.
Since
 , therefore g(x) has a greater maximum value than f(x).
, therefore g(x) has a greater maximum value than f(x).
Hence, the correct option is C.