Question

Small Sample:

During an economic downturn, 11 companies were sampled and asked whether they were planning to increase their workforce. Only 2 of the 11 companies were planning to increase their workforce. Use the small-sample method to construct an 80% confidence interval for the proportion of companies that are planning to increase their workforce. A pollster wants to construct a 95% confidence interval for the proportion of adults who believe that economic conditions are getting better. A poll taken in July 2010 estimates this proportion to be 0.36. Using this estimate, what sample size is needed so that the confidence interval will have a margin of error of 0.01?

Answer 1

The 80% confidence interval for the proportion of companies that are planning to increase their workforce is (0.033, 0.331).

A sample size of 8852 is needed.

Explanation:

First question:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

In which

z is the zscore that has a pvalue of
`1 - (\alpha)/(2)`.

Only 2 of the 11 companies were planning to increase their workforce.

This means that
`n = 11, \pi = (2)/(11) = 0.1818`

80% confidence level

So
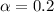`\alpha = 0.2`, z is the value of Z that has a pvalue of
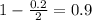`1 - (0.2)/(2) = 0.9`, so
`Z = 1.28`.

The lower limit of this interval is:

`\pi - z\sqrt{(\pi(1-\pi))/(n)} = 0.1818 - 1.28\sqrt{(0.1818*0.8182)/(11)} = 0.033`

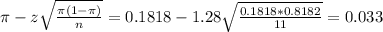
The upper limit of this interval is:

`\pi + z\sqrt{(\pi(1-\pi))/(n)} = 0.1818 + 1.28\sqrt{(0.1818*0.8182)/(11)} = 0.331`

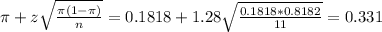
The 80% confidence interval for the proportion of companies that are planning to increase their workforce is (0.033, 0.331).

Second question:

The margin of error is:

`M = z\sqrt{(\pi(1-\pi))/(n)}`

A poll taken in July 2010 estimates this proportion to be 0.36.

This means that
`\pi = 0.36`

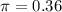
95% confidence level

So
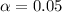`\alpha = 0.05`, z is the value of Z that has a pvalue of
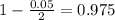`1 - (0.05)/(2) = 0.975`, so
`Z = 1.96`.

Using this estimate, what sample size is needed so that the confidence interval will have a margin of error of 0.01?

This is n for which M = 0.01. So

`M = z\sqrt{(\pi(1-\pi))/(n)}`

`0.01 = 1.96\sqrt{(0.36*0.64)/(n)}`

`0.01√(n) = 1.96√(0.36*0.64)`

`√(n) = (1.96√(0.36*0.64))/(0.01)`

`(√(n))^2 = ((1.96√(0.36*0.64))/(0.01))^2`

`n = 8851.04`

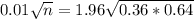
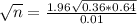
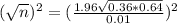
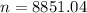
Rounding up(as a sample of 8851 will have a margin of error slightly over 0.01):

A sample size of 8852 is needed.