Question

Of the students living in the dormitories at aa€‹ university, 65% live at the westa€‹ hall, and the rest at the south tower. A sandwich shop randomly mails a coupon for a free sandwich to 24% of those at the westa€‹ hall, and to 19% of those living at the south tower. Let D be the event that the person receive a coupon, E1 be the event that the person is living in the westa€‹ hall, and E2 be the event that the person is living in the south tower. A student living in a dormitory is randomly chosen.

Required:
Find the probability that this person received a coupon. Also write an expression that models the probability.

Answer 1

The answer is "Option A".

Explanation:

Dormitory students.

Please find the complete question in the attched file.

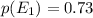`p (E_1) =0.73`indicates that teachers live in the west hall.

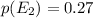`p (E_2) =0.27` indicates that the students live in the south tower.

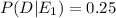`P (D|E_1) =0.25` describes the likelihood which West Hall students received free coupons.

`P (D|E_2) =0.19` indicates that students in the south tower were given free coupons.

`P(D)=P(D|E_1)P(E_1)+P(D|E_2)P(E_2)\\\\=0.25 * 0.73 +0.19 * 0.27\\\\=0.234`

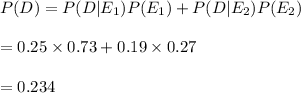
To maximize the probability that the person selected at random will receive the coupon, multiply the following numbers by the possibility of success of the coupon: