Question

a right triangle has one leg that’s 5 units long and a hypotenuse that’s 8 units long. how long is the other leg?

Answer 1

We are given that , in a right angled triangle the hypotenuse is 8 units and it's one leg is 5 units . And we need to find the another leg . So , here Pythagoras theorem will be very helpful for us which states that in any Right Triangle , the sum of square of it's two sides ( base and perpendicular or two legs ) is equal to the square of it's largest side ( Hypotenuse )

Now , let's assume that the other leg be x , so now by Pythagoras theorem ;

`{:\implies \quad \sf x^(2)+5^(2)=8^(2)}`

`{:\implies \quad \sf x^(2)+25=64}`

`{:\implies \quad \sf x^(2)=64-25}`

`{:\implies \quad \sf x^(2)=39}`

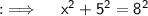
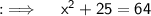
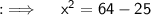
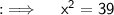
Raising power to ½ on both sides will leave us with x = +√39 , -√39. But as length can never be -ve

`{:\implies \quad \bf \therefore \underline{\underline{x=√(39)\:\: units}}}`

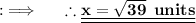
Hence , the another leg of the right angled triangle is √39 units

Answer 2

• Given - a right triangle with length of one side = 5 units and with hypotenuse of length = 8 units.

By applying Pythagoras theorem ,

`h {}^(2) = p {}^(2) + b {}^(2) \\ (8) {}^(2) = (5) {}^(2) + b {}^(2) \\ 64 = 25 + b {}^(2) \\ b {}^(2) = 64 - 25 \\ b {}^(2) = 39 \\ b = √(39 \:) \: units`

hope helpful~