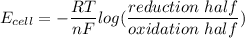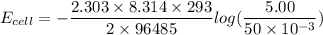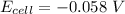Question

A certain metal M forms a soluble sulfate salt M2SO4. Suppose the left half cell of a galvanic cell apparatus is filled with a 50.0 mM solution of M2SO4 and the right half cell with a 5.00 M solution of the same substance. Electrodes made of M are dipped into both solutions and a voltmeter is connected between them The temperature of the apparatus is held constant at 20.0 °C.

Which electrode will be positive?
What voltage will the voltmeter show?

Answer 1

Step-by-step explanation:

For a certain metal M by which the electrodes and the solution M_2SO_4 the cell notation is:

SALT BRIDGE

ANODE ↓ CATHODE

`M(s)|M_2SO_4_((aq)) (50.0 \ mM)\Big \Big M_2SO_4_((aq)) (5.00 \ M ) |M(s)`

Since the standard electrode potential is less positive on the left side, a negative anode electrode is used, and oxidation occurs at the anode.

`i.e \ \ M(s) \to M^(2+) _((aq)) + 2 e^- \ \ \ (oxidation)`

The right side of the cell cathode is placed which is positive in sign and aids in reduction since the normal reduction potential is less negative.

`i.e \ \ M^(2+) _((aq)) + 2 e^- \to M(s) \ \ \ (reduction)`

As a result, the correct answer for the positive electrode is the right side.

`M_2SO_4 \to M^(2+) + SO_4^(2-)`

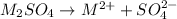
Using Nernst Equation:

`E_(cell) = - (RT)/(nF )log ( (reduction \ half )/(oxidation \ half))`

`E_(cell) = - (2.303 * 8.314 * 293)/(2 * 96485 )log ( (5.0 0 )/(50 * 10^(-3)))`

`E_(cell) = -0.058 \ V`