Answer:
The magnitude and direction of the resultant force are approximately 599.923 newtons and 36.405°.
Step-by-step explanation:
First, we must calculate the resultant force (
 ), in newtons, by vectorial sum:
), in newtons, by vectorial sum:
![\vec F = [(-200\,N)\cdot \cos 60^(\circ)+(400\,N)\cdot \cos 45^(\circ)+300\,N]\,\hat{i} + [(200\,N)\cdot \sin 60^(\circ) + (400\,N)\cdot \sin 45^(\circ)-100\,N]\,\hat{j}](https://img.qammunity.org/2022/formulas/physics/high-school/7wmutn7jxlwnll4wakwnais7v4fh947l2l.png) (1)
(1)

Second, we calculate the magnitude of the resultant force by Pythagorean Theorem:
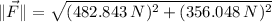
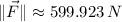
Let suppose that direction of the resultant force is an standard angle. According to (1), the resultant force is set in the first quadrant:

Where
 is the direction of the resultant force, in sexagesimal degrees.
is the direction of the resultant force, in sexagesimal degrees.

The magnitude and direction of the resultant force are approximately 599.923 newtons and 36.405°.