Question

Roll the number cube to find the observed frequency. Which statements are true? Check all that apply

Answer 1

Person above/under me is incorrect

Explanation:

Answer is A and D Because i got their answer wrong

Answer 2

(a) and (c)

Explanation:

Given

See attachment for complete question

Required

Select all true statements

First, calculate the total observed frequency;

`Total = 11 + 16 + 14 + 20 + 12 + 17`

`Total = 90`

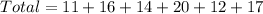
(a): Relative frequency of 4 is 2/9

Relative frequency is calculated by:

`RF= (Observed\ Frequency)/(Total)`

For roll 4

`Observed\ frequency = 20`

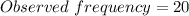
So, the relative frequency is:

`RF = (20)/(90)`

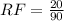
Simplify

`RF = (2)/(9)`

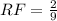
(a) is true

(b): Experimental probability of 3 greater than its theoretical probability

The experimental probability is its relative frequency;

For roll 3

`Observed\ frequency = 14`

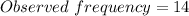
The experimental probability is:

`Pr = (14)/(90)`

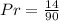
Simplify

`Pr = (7)/(45) = 0.156`

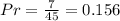
To calculate the theoretical probability, w have:

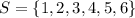`S = \{1,2,3,4,5,6\}` ---- sample space

`n(S)= 6`

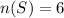
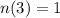`n(3) = 1` --- number of times 3 occur

The theoretical probability is:

`Pr = (1)/(6) = 0.167`

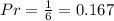
The theoretical probability is
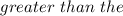`greater\ than\ the` experimental probability.

(b) is false

(c): Experimental probability of 2 greater than its theoretical probability

Using the explanations in (b), we have:

For roll 2

`Observed\ frequency = 16`

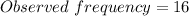
The experimental probability is:

`Pr = (16)/(90)`

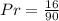
Simplify

`Pr = (8)/(45) = 0.178`

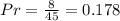
To calculate the theoretical probability, we have:

`n(S)= 6`

`n(2) = 1`

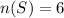
The theoretical probability is:

`Pr = (1)/(6) = 0.167`

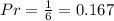
The experimental probability is
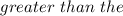`greater\ than\ the` theoretical probability.

(c) is true

(d): Relative frequency of 5 is 2/13

For roll 5

`Observed\ frequency = 12`

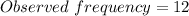
The relative frequency is:

`RF = (12)/(90)`

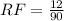
Simplify

`RF = (2)/(15)`

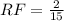
(d) is true