Question

In 1995, 13,000 Internet users were surveyed and asked about their willingness to pay fees for access to websites. Of these, 3,250 were definitely not willing to pay such fees. Construct a 95 percent confidence interval for the proportion definitely unwilling to pay fees. [0.243, 0.257] [0.212, 0.241] [0.286, 0.302] [0.219, 0.233] [0.214, 0.245]

Answer 1

[0.243, 0.257]

Explanation:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

In which

z is the zscore that has a pvalue of
`1 - (\alpha)/(2)`.

In 1995, 13,000 Internet users were surveyed and asked about their willingness to pay fees for access to websites. Of these, 3,250 were definitely not willing to pay such fees.

This means that
`n = 13000, \pi = (3250)/(13000) = 0.25`

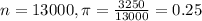
95% confidence level

So
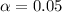`\alpha = 0.05`, z is the value of Z that has a pvalue of
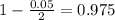`1 - (0.05)/(2) = 0.975`, so
`Z = 1.96`.

The lower limit of this interval is:

`\pi - z\sqrt{(\pi(1-\pi))/(n)} = 0.25 - 1.96\sqrt{(0.25*0.75)/(13000)} = 0.243`

The upper limit of this interval is:

`\pi + z\sqrt{(\pi(1-\pi))/(n)} = 0.25 + 1.96\sqrt{(0.25*0.75)/(13000)} = 0.257`

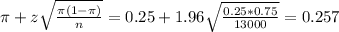
The answer is [0.243, 0.257]