Answer:
72
Explanation:
One way to do this is to use the formula
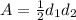 where
where
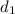 and
and
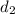 are the lengths of the two diagonals.
are the lengths of the two diagonals.
In the figure,
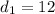 and
and
 (2 + 10 and 6 + 6).
(2 + 10 and 6 + 6).
The area is
 square units.
square units.
Another way that doesn't depend on learning a formula is to remember that the diagonals of a kite are perpendicular, so the 4 small triangles are right triangles. Two of the smaller triangles have base =2 and height = 6, so their areas are 1/2(2)(6) = 6. Double that--there are 2 congruent small triangles--to get an area of 12.
There are two larger right triangles with base =10 and height = 6, so their area are 1/2(10)(6)=30. There are two of those, so their combined area is 60.
Finally, put the small triangle area and the large triangle area together to get a total of 12 + 60 = 72