Question

The times when goals are scored in hockey aremodeled as a Poisson process inMorrison (1976). For such a process, assume that the average time between goals is 15 minutes. (i) In a 60-minute game, find the probability that a fourth goal occurs in the last 5 minutes of the game. (ii) Assume that at least three goals are scored in a game. What is the mean time of the third goal

Answer 1

This question is incomplete, the complete question is;

The times when goals are scored in hockey are modeled as a Poisson process in Morrison (1976). For such a process, assume that the average time between goals is 15 minutes.

(The parameter of the hockey Poisson Process is lambda = 1/15 )

(i) In a 60-minute game, find the probability that a fourth goal occurs in the last 5 minutes of the game?

(ii) Assume that at least three goals are scored in a game. What is the mean time of the third goal?

Answer:

i) the probability that a fourth goal occurs in the last 5 minutes of the game is 0.068

ii) The mean time of the third goal is 33.5 minutes

Explanation:

Given the data in the question;

The parameter of the hockey Poisson Process λ = 1/15

i)

Let us represent the probability of a fourth goal in the last 5 min in a 60 min game with X.

Thus, we find the probability that X is greater than ( 60min - 5min) and less than or equal to 60min

so;

p( 55 < X ≤ 60 ) =
`(1)/(6) \int\limits^(60)_(55) ((1)/(15))^4 ( t^3) (e^(-t)) dt`

p( 55 < X ≤ 60 ) = 0.06766 ≈ 0.068

Therefore, the probability that a fourth goal occurs in the last 5 minutes of the game is 0.068

ii)

Also let us represent the probability that at least 3 goals are scored in the game with X

Now, the mean time of the third goal will be;

P(X|X < 60 ) =
`(1)/(P(X<60)) \int\limits^0_(60) t((1/15)^3 (t^2) ( e^(-t/15)) )/(2) dt`

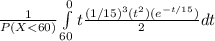
P(X|X < 60 ) = 25.49 / 0.76

P(X|X < 60 ) = 33.539 ≈ 33.5

Therefore, the mean time of the third goal is 33.5 minutes