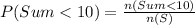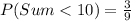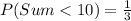Answer:

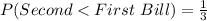
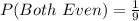
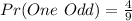
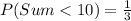
Explanation:
Given

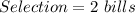
The sample space is as follows:
This implies that we construct possible outcome that Ebony selects a bill, returns the bill and then select another.
This means that there are possibilities that the same bill is selected twice.
So, the sample space is as follows:
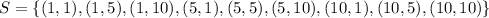
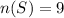
Solving (a):
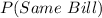
This means that the first and second bill selected are the same.
The outcome of this are:

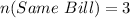
The probability is:
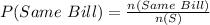


Solving (a):

This means that the second bill selected is less than the first.
The outcome of this are:
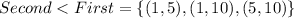
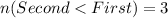
The probability is:
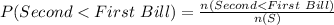
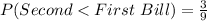
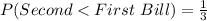
Solving (c):
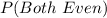
This means that the first and the second bill are even
The outcome of this are:
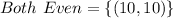
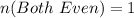
The probability is:
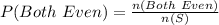
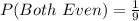
Solving (e):
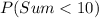
This question has missing details.
The correct question is to determine the probability that, the sum of both bills is less than 10
The outcome of this are:
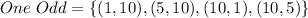
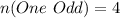
The probability is:

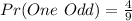
Solving (d):
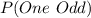
This question has missing details.
The correct question is to determine the probability that, exactly one of the bills is 0dd
The outcome of this are:
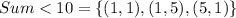
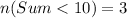
The probability is: