Answer:
The answer to each question is:
- A.) 403.2
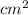
- B.) 100. 8
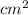
Explanation:
A.) To obtain the area of the dodecagon, you can use the next formula:
- Area of a dodecagon = 6 * apothem * edge
In the exercise, we have the edge (6 cm), but, to find the apothem of a dodecagon, that is the distance between the center of the polygon, and the middle point of each side, you can use the next formula:
- Apothem = Edge *
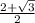
If we replace the value of the edge, we obtain:
- Apothem = 6 cm *
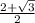
- Apothem = 11.2 cm (approximately).
With this data, we can find the area:
- Area of a dodecagon = 6 * apothem * edge
- Area of a dodecagon = 6 * 11.2 cm * 6 cm
- Area of a dodecagon = 403.2
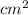
Then, the area of the dodecagon is 403.2
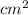 approximately.
approximately.
B.) As the area obtained is for 12 pieces, when the parents ate 9 pieces, just left 3 pieces, with these values you can make a rule of three.
If:
- 12 pieces = 403.2
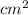
- 3 pieces = X
Then:
- X = (3 * 403.2
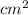 ) / 12
) / 12 - X = 1209.6
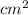 / 12
/ 12 - X = 100. 8
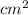
In this form, when the cookie has 3 pieces, its area is 100. 8
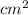 approximately.
approximately.