Consider the below figure attached with this question.
Given:
A, B & C lie on a straight line. D, C & E lie on a different straight line.
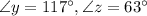
To find:
The value of x.
Solution:
A, B & C lie on a straight line.
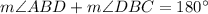 (Linear pair)
(Linear pair)
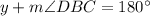
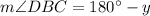
According to the exterior angle theorem, the measure of an exterior angle of a triangle is equal to the sum of two opposite interior angles.
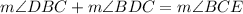 (Exterior angle theorem)
(Exterior angle theorem)
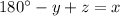
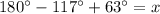

Therefore, the value of x is 126 degrees.