Answer:
μ = tan θ
Step-by-step explanation:
For this exercise let's use the translational equilibrium condition.
Let's set a datum with the x axis parallel to the plane and the y axis perpendicular to the plane.
Let's break down the weight of the block
sin θ = Wₓ / W
cos θ = W_y / W
Wₓ = W sin θ
W_y = W cos θ
The acrobat is vertically so his weight decomposition is
sin θ = = wₐₓ / wₐ
cos θ = wₐ_y / wₐ
wₐₓ = wₐ sin θ
wₐ_y = wₐ cos θ
let's write the equilibrium equations
Y axis
N- W_y - wₐ_y = 0
N = W cos θ + wₐ cos θ
X axis
Wₓ + wₐ_x - fr = 0
fr = W sin θ + wₐ sin θ
the friction force has the formula
fr = μ N
fr = μ (W cos θ + wₐ cos θ)
we substitute
μ (Mg cos θ + mg cos θ) = Mgsin θ + mg sin θ
μ =
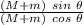
μ = tan θ
this is the minimum value of the coefficient of static friction for which the system is in equilibrium.