Answer:
The distance between Mary's house and her grandmother's house is approximately 49.73 miles.
The 3 traveled the following distances:
Theo rode 50 miles, Mary drove 49.73 miles, and Nancy drove 53.299 miles.
Explanation:
Mary's route is indeed the hypotenuse of a given triangle, that has a 13-mile adjacent hand and a 48-mile wrong side. They can solve for the length of the other side of the triangle using Pythagoras' theorem because we have two sides of the triangle.
Let us just say that triangle's ellipse is x square miles. Pythagoras's theory stated that
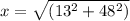
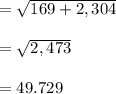
Mary's route was 49.73 square miles, to sum it up.
Nancy drove
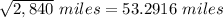
As a result, Mary rode the shortest distance of 49.73 miles, Theo drove 50 miles, and Nancy drove 53.299 miles.