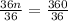Question

Hello there,

can someone please help me with this question

Answer 1

10 sides.

Explanation:

Two same interior angles + 72 degrees = 360.

Thus, the interior angle of the polygon is
`(360-72)/(2) =144`.

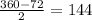The formula to find the interior angle of a polygon is
`(180(n-2))/(n)`

`(180(n-2))/(n) =144`

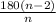
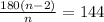
Multiply both sides by n:

`(180\left(n-2\right))/(n)n=144n`

`180\left(n-2\right)=144n`

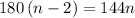
Expand:

`180n-360=144n`

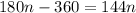
Add 360 to both sides:

`180n-360+360=144n+360`

`180n=144n+360`

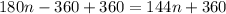
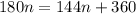
Subtract 144n from both sides:

`180n-144n=144n+360-144n`

`36n=360`

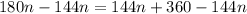
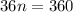
Divide both sides by 36:

`(36n)/(36)=(360)/(36)`

`n=10`