Question

You want to find out how many atoms of the isotope 65Cu are in a small sample of material. You bombard the sample with neutrons to ensure that on the order of 1% of these copper nuclei absorb a neutron. After activation, you turn off the neutron flux and then use a highly efficient detector to monitor the gamma radiation that comes out of the sample. Assume half of the 66Cu nuclei emit a 1.04-MeV gamma ray in their decay. (The other half of the activated nuclei decay directly to the ground state of 66Ni.) (Enter your answer using one of the following formats: 1.2e-3 for 0.0012 and 1.20e 2 for 120.)

Required:
a. If after 10 min (two half-lives) you have detected 10000 MeV of photon energy at 1.04 MeV, approximately how many 65Cu atoms are in the sample?
b. Assume the sample contains natural copper. Refer to the isotopic abundances listed in your text (Chemical and Nuclear Information for Selected Isotopes) and estimate the total mass of copper in the sample.

Answer 1

a) number of copper atoms 65 (⁶⁵Cu) is 7.692 10⁶ atoms

b) m_total Cu = 1.585 10⁹ u = 2.632 10⁻¹⁸ kg

Step-by-step explanation:

a) For this exercise let's start by using the radioactive decay ratio

N = N₀
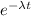`e^(- \lambda t)`o e - lambda t

The half-life time is defined as the time it takes for half of the radioactive (activated) atoms to decay, therefore after two half-lives there are

N = ½ (½ N₀) = ¼ N₀

N₀ = 4 N

in each decay a photon is emitted so we can use a direct rule of proportions. If an atom emits a photon it has Eo = 1,04 Mev, how many photons it has energy E = 10,000 MeV

# _atoms = 1 atom (photon) (E / Eo)

# _atoms = 1 10000 / 1.04

# _atoms = 9615,4 atoms

N₀ = 4 #_atoms

N₀ = 4 9615,4

N₀= 38461.6 atoms

in the exercise indicates that half of the atoms decay in this way and the other half decays directly to the base state of Zinc, so the total number of activated atoms

N_activated = 2 # _atoms

N_activated = 2 38461.6

N_activated = 76923.2

also indicates that 1% = 0.01 of the nuclei is activated by neutron bombardment

N_activated = 0.01 N_total

N_total = N_activated / 0.01

N_total = 76923.2 / 100

N_total = 7.692 10⁶ atoms

so the number of copper atoms 65 (⁶⁵Cu) is 7.692 10⁶

b) the natural abundance of copper is

⁶³Cu 69.17%

⁶⁵Cu 30.83%

Let's use a direct proportion rule. If there are 7.692 10⁶ ⁶⁵Cu that represents 30.83, how much ⁶³Cu is there that represents 69.17%

# _63Cu = 69.17% (7.692 10⁶ / 30.83%)

# _63Cu = 17.258 10⁶ atom ⁶³Cu

the total amount of comatose is

#_total Cu = #_ 65Cu + # _63Cu

#_total Cu = (7.692 + 17.258) 10⁶

#_total Cu = 24.95 10⁶

the atomic mass of copper is m_Cu = 63.546 u

m_total = #_totalCu m_Cu

m_total = 24.95 10⁶ 63,546 u

m_total = 1.585 10⁹ u

let's reduce to kg

m_total Cu = 1.585 10⁹ u (1,66054 10⁻²⁷ kg / 1 u)

m_total Cu = 2.632 10⁻¹⁸ kg