Question

A wooden board is leaning against a wall. The base of the board is 10 feet from the base of the wall. The base of the board forms a 35° angle with the ground.

What is the length of the wooden board?

Round your answer to nearest tenth.

Answer 1

The length of the wooden board is about 12.2 feet.

Explanation:

Please refer to the diagram attached below.

The base of the board forms a 35° degree angle with the ground.

And we want to find the length of the wooden board.

Since we are given an angle and its adjacent length and we want to find the hypotenuse, we can use the cosine ratio:

`\displaystyle \cos(\theta^\circ)=\frac{\text{adjacent}}{\text{hypotenuse}}`

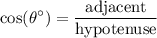
The adjacent side is the distance from the wall to the board, which is 10 feet.

And the angle made is 35°.

The hypotenuse is the length of the wooden board. Therefore:

`\displaystyle \cos(35)=(10)/(\ell)`

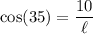
Solve for the length. Cross-multiply:

`\displaystyle 10=\ell \cos(35)`

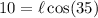
Therefore:

`\displaystyle \ell=(10)/(\cos(35))\approx12.2`

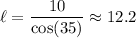
The length of the wooden board is about 12.2 feet.