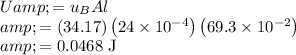Question

A solenoid that is 69.3 cm long has a cross-sectional area of 24.0 cm2. There are 1260 turns of wire carrying a current of 8.78 A. (a) Calculate the energy density of the magnetic field inside the solenoid. (b) Find the total energy in joules stored in the magnetic field there (neglect end effects).

Answer 1

a) 34.17J/m^3

b) 0.0468 J

Step-by-step explanation:

a) Calculate the energy density of the magnetic field inside the solenoid as follows:

`u_(B) &=(U)/(A l) \\ &=(L I^(2))/(2 A l) \quad\left(U=(1)/(2) L I^(2)\right) \\ &=(\mu_(0) N^(2) A I^(2))/(2 A l^(2)) \quad\left(L=(\mu_(0) N^(2) A)/(l)\right) \\ &=(\mu_(0) N^(2) I^(2))/(2 l^(2))`

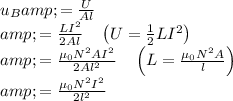
Substitute the corresponding values in the above equation.

`u_(B) &=(4 \pi\left(10^(-7)\right)(1260)^(2)(8.78)^(2))/(2(0.693)^(2)) \\</p><p>&=34.17 \mathrm{~J} / \mathrm{m}^(3)`

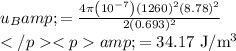
b) Calculate the total energy stored in the solenoid as follows:

`U &=u_(B) A l \\ &=(34.17)\left(24 * 10^(-4)\right)\left(69.3 * 10^(-2)\right) \\ &=0.0468 \mathrm{~J}`