Question

What is the length of side x in a 30-60-90 triangle where one side is the square root of 3 and the other side is unknown?

Answer 1

The length of side x in a 30-60-90 triangle is 2√3.

Explanation:

The numbers 30-60-90 are angles, so we need to find the side x of a right triangle with the following information:

θ: is one angle of the right triangle = 30°

α: is the other angle of the right triangle = 60°

a: is one side of the right triangle = √3

b: is the other side of the right triangle =?

x: is the hypotenuse of the right triangle =?

The length of the hypotenuse can be found by Pitagoras:

`x^(2) = a^(2) + b^(2)` (1)

So, we need to find the side "b". We can calculate it with the given angles.

From the side "a" we have:

`cos(\alpha) = (a)/(x)`

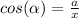
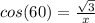`cos(60) = (√(3))/(x)` (2)

From the side "b":

`sin(\alpha) = (b)/(x)`

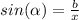
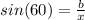`sin(60) = (b)/(x)` (3)

Now, we can calculate "b" by dividing equation (3) by equation (2).

`tan(60) = ((b)/(x))/((√(3))/(x))`

`b = tan(60)*√(3) = 3`

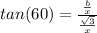
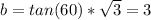
Finally, we can find the length of the hypotenuse with equation (1):

`x = \sqrt{a^(2) + b^(2)} = \sqrt{(√(3))^(2) + (3)^(2)} = 2√(3)`

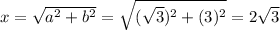
Therefore, the length of side x in a 30-60-90 triangle is 2√3.

I hope it helps you!