Question

In the triangle shown below, find the value of a.

45°
9.2
I don’t get this someone pleas help out!

Answer 1

Given:

The figure of a right angle triangle.

Measure of one angle is 45 degrees.

Hypotenuse =
`9√(2)`

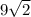
Opposite side of angle 45 degrees = a

To find:

The value of a.

Solution:

In a right angle triangle,

`\sin \theta=(Perpendicular)/(Hypotenuse)`

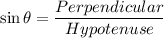
It can be rewritten as:

`\sin \theta=(Opposite)/(Hypotenuse)`

For the given triangle,

`\sin (45^\circ)=(a)/(9√(2))`

`(1)/(√(2))=(a)/(9√(2))`

`(9√(2))/(√(2))=a`

`9=a`

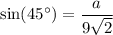
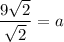
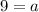
Therefore, the value of a is 9 units.