Answer:
cos²Θ
Explanation:
simplify the expression using the identities
tanΘ =
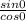
cos2Θ = 1 - 2sin²Θ
cos²Θ = 1 - sin²Θ
then
tanΘ × sinΘ × cosΘ + cos2Θ
=
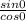 × sinΘ × cosΘ + 1 - 2sin²Θ ( cancel cosΘ on numerator/ denominator )
× sinΘ × cosΘ + 1 - 2sin²Θ ( cancel cosΘ on numerator/ denominator )
= sinΘ × sinΘ + 1 - 2sin²Θ
= sin²Θ + 1 - 2sin²Θ
= 1 - sin²Θ
= cos²Θ