Answer:
The expected mass of the sample in the year 2009 is approximately 60 miligrams.
Explanation:
The mass of radioactive isotopes decays exponentially in time, the equation that represents this phenomenon is presented below:
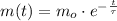 (1)
(1)
Where:
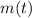 - Current mass of the radioactive isotope, in miligrams.
- Current mass of the radioactive isotope, in miligrams.
 - Initial mass of the radioactive isotope, in miligrams.
- Initial mass of the radioactive isotope, in miligrams.
 - Time, in years.
- Time, in years.
 - Time constant, in years.
- Time constant, in years.
First, we must find the time constant associated to the decay of the radioactive isotope by clearing the respective term in (1):

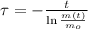
If we know that
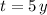 ,
,
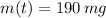 and
and
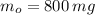 , then the time constant is:
, then the time constant is:
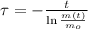
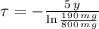
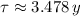
Now, we calculate the mass of the radioactive isotope in 2009 (
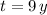 ). If we know that
). If we know that
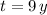 ,
,
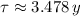 and
and
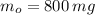 , then the mass of the radioactive isotope is:
, then the mass of the radioactive isotope is:
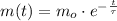
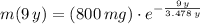
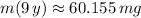
The expected mass of the sample in the year 2009 is approximately 60 miligrams.