Question

If cos 0 = 3/radical 22 and angle 0 is in Quadrant I, what is the exact value of tan 20 in

simplest radical form?

Answer 1

• 
  `\tan2\theta =-(3√(13))/(2)`

Explanation:

To find:-

• The value of
  `\tan2\theta` .

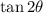Answer:-

We are here given that the value of,

`\longrightarrow \cos\theta=(3)/(√(22)) \\`

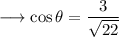
And we know that cosine is the ratio of base and hypotenuse. Therefore,

`\longrightarrow \cos\theta =(b)/(h) \\`

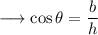
So we can also say that,

`\longrightarrow \cos\theta = (b)/(h)=(3)/(√(22))\\`

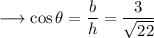
Let us take the given ratio to be 3x:√22x . Now we may find out the value of perpendicular using Pythagoras theorem . The Pythagoras theorem is ,

Pythagoras theorem:-

• In a right angled triangle the sum of squares of perpendicular and base is equal to the square of hypotenuse.

Mathematically,

`\longrightarrow \boxed{ \boldsymbol{p^2+b^2=h^2}} \\`

where the symbols have their usual meaning.

On substituting the respective values, we have;

`\longrightarrow p^2+(3x)^2=(√(22)x)^2 \\`

`\longrightarrow p^2 = 22x^2-9x^2\\`

`\longrightarrow p^2=13x^2 \\`

`\longrightarrow \boldsymbol{ p = √(13)x} \\`

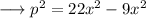
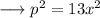
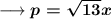
Now again , we know that, tangent is defined as the ratio of perpendicular and base. Therefore,

`\longrightarrow \tan\theta = (p)/(b) \\`

`\longrightarrow \tan\theta = (√(13)x)/(3x)\\`

`\longrightarrow \boldsymbol{ \tan\theta = (√(13))/(3)}\\`

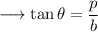
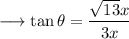
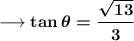
Now we may use a identity which is ,

`\longrightarrow\boxed{ \boldsymbol{ \tan (2x)= (2\tan x)/(1-\tan^2 x)}}\\`

On substituting the respective values, we have;

`\longrightarrow \tan2\theta = ( 2\tan\theta)/(1- \tan^2\theta)\\`

`\longrightarrow \tan2\theta = (2\bigg((√(13))/(3)\bigg))/(1-\bigg((√(13))/(3)\bigg)^2 ) \\`

`\longrightarrow \tan2\theta = ((2√(13))/(3))/(1-(13)/(9)) \\`

`\longrightarrow \tan2\theta = ((2√(13))/(3))/((9-13)/(9)) \\`

`\longrightarrow \tan2\theta = ((2√(13))/(3))/((-4)/(9)) \\`

`\longrightarrow \tan2\theta = -(2√(13)\cdot 9)/(3\cdot 4 ) \\`

`\longrightarrow \boxed{\boldsymbol{ \tan2\theta = -(3√(13))/(2)}} \\`

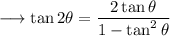
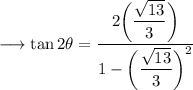
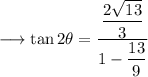
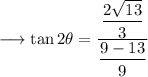
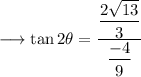
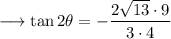
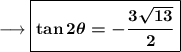
This is the required answer .