Question

A 1.0-kg object moving 9.0 m/s collides with a 2.0-kg object moving 6.0 m/s in a direction that is perpendicular to the initial direction of motion of the 1.0-kg object. The two masses remain together after the collision, and this composite object then collides with and sticks to a 3.0-kg object. After these collisions, the final composite (6.0-kg) object remains at rest. What was the speed of the 3.0-kg object before the collisions

Answer 1

v₃ = - (3 i ^ + 4 j ^) m / s

v₃ = 5 m / s, θ = 233º

Step-by-step explanation:

This is a momentum problem. Let us form a system formed by the three objects so that the forces during the collisions have been internal and the moment is conserved.

Let's start working with the first two objects. As each object moves in a different direction let's work with the components in an xy coordinate system

X axis

initial instant. Before the shock

p₀ₓ = m₁ v₁₀ + 0

final instant. After the crash

p_{fx} = (m1 + m2) vₓ

the moment is preserved

p₀ₓ = p_{fx}

m₁ v₀₁ = (m₁ + m₂) vₓ

vₓ =
`(m_1)/(m_1+m_2) \ v_(o1)`

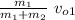
Y axis

initial instant

p_{oy} = 0 + m₂ v₀₂

final moment

p_{fy} = (m₁ + m₂) v_y

the moment is preserved

p_{oy} = p_{fy}

m₂ v₀₂ = (m₁ + m₂) v_y

v_y =
`(m_2)/(m_1 +m_2 ) \ v_(o2)`

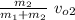
We already have the speed of the set of these two cars, now let's work on this set and vehicle 3

X axis

initial instant

p₀ₓ = (m₁ + m₂) vₓ + m₃ v₃ₓ

final instant

p_{fx} = 0

p₀ₓ = p_{fx}

(m₁ + m₂) vₓ + m₃ v₃ₓ = 0

v₃ₓ =
`- (m_1+m_2 )/(m_3) \ v_x`

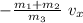
Y Axis

initial instant

p_{oy} = (m₁ + m₂) v_y + m₃ v_{3y}

final moment

p_{fy} = 0

p_{oy} = p_{fy}

(m₁ + m₂) v_y + m₃ v_{3y} = 0

v_{3y} =
`- (m_1+m_2)/(m_3) \ v_y`

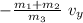
now we substitute the values ​​of the speeds

v₃ₓ =
`- (m_1+m_2)/(m_3) \ (m_1)/(m_1+m_2) \ v_(o1)`

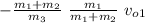
v₃ₓ =
`- (m_1)/(m_3) \ v_(o1)`

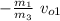
v_{3y} =
`- (m_1+m_2)/(m_3) \ (m_2)/(m_1+m_2) \ v_(o2)`

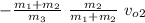
v_{3y} =
`- (m_2)/(m_3) \ v_(o2)`

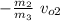
let's calculate

v₃ₓ = - ⅓ 9

v₃ₓ = - 3 m / s

v_{3y} = - ⅔ 6

v_{3y} = - 4 m / s

therefore the speed of vehicle 3 is

v₃ = - (3 i ^ + 4 j ^) m / s

It can also be given in the form of modulus and angles using the Pythagorean theorem

v₃ =
`\sqrt{v_(3x)^2 + v_(3y)^2}`

v₃ =
`√(3^2+4^2)`

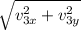
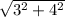
v₃ = 5 m / s

let's use trigonometry for the angle

tan θ' =
`(v_(3y))/(v_(3x))`

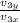
θ' = tan⁻¹ (\frac{v_{3y}}{v_{3x}})

θ' = tan⁻¹ (4/3)

θ' = 53º

That the two speeds are negative so this angle is in the third quadrant, measured from the positive side of the x axis

θ = 180 + θ'

θ = 180 +53

θ = 233º