Given:
One diagonal of the kite is three times as long as the other diagonal.
The area of the kite is 48 square meters.
To find:
The length of each diagonal.
Solution:
We know that, the area of the kite is:
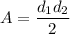
Where,
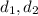 are two diagonals of a kite.
are two diagonals of a kite.
It is given that, one diagonal of the kite is three times as long as the other diagonal.
Let
 . Then the area of the kite is:
. Then the area of the kite is:
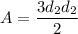
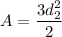
The area of the kite is 48 square meters.
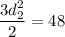
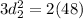
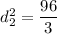
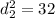
Taking square root on both sides, we get
 [It is only positive because side length cannot be negative]
[It is only positive because side length cannot be negative]
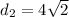
Now,

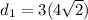
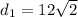
Therefore, the diagonals of the kite are
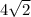 units and
units and
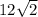 units.
units.