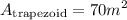Question

Find the area of this trapezoid. Include the correct unit in your answer.

I need help understanding this and how to do it :)​

Answer 1

`\displaystyle A _{ \text{trapezoid}} = 70 {m}^(2)`

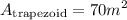
Explanation:

we are given a trapezoid

we want to figure out the area

remember that,

`\displaystyle A _{ \text{trapezoid}} = (a + b)/(2) h`

where a and b represent the parallel lines and h represents the height

we get from the pic that a and b are 5 and 15 respectively and h is 7

so substitute:

`\displaystyle A _{ \text{trapezoid}} = (5 + 15)/(2) * 7`

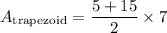
simplify addition:

`\displaystyle A _{ \text{trapezoid}} = (20)/(2) * 7`

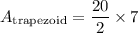
simplify division:

`\displaystyle A _{ \text{trapezoid}} = 10* 7`

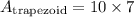
simplify multiplication:

`\displaystyle A _{ \text{trapezoid}} = 70`

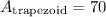
since we multiply two same units we of course have to use square unit

hence,

`\displaystyle A _{ \text{trapezoid}} = 70 {m}^(2)`