Question

Select the correct function. Select the function with an average rate of change of 3 over the interval [1, 3].

Answer 1

Function g(x)

Explanation:

Given

See attachment for functions

Required

Which has an average rate of 3 over [1,3]

The average rate of change (m) is calculated as:

`m = (f(b) - f(a))/(b -a)`

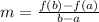
Where:

`[a,b] = [1,3]`

So, we have:

`m = (f(3) - f(1))/(3 -1)`

`m = (f(3) - f(1))/(2)`

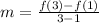
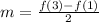
From the table f(x), we have:

`f(3) = 6\\ f(1) = -2`

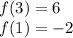
So:

`m = (6 - -2)/(2)`

`m = (8)/(2)`

`m =4`

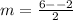
From the graph of g(x), we have:

`g(3) = 4\\ g(1) = -2`

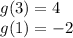
So:

`m = (g(3) - g(1))/(2)`

`m = (4 - -2)/(2)`

`m = (6)/(2)`

`m =3`

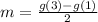
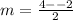
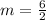
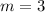
Since only one of the function has an average rate of change of 3 over the given interval,

Then g(x) answers the question