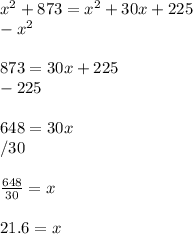Question

Please show all work

Answer 1

`x=21.6`

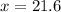
Explanation:

Use the Pythagorean theorem to solve this problem. The Pythagorean theorem states the following,

`a^2 + b^c = c^2`

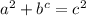
Where (a) and (b) are the legs or the sides adjacent to the right angle, and (c) is the hypotenuse or the side opposite the right angle.

Use the Pythagorean theorem in the right triangle with legs (15) and (18),

`(15)^2+(18)^2=c^2\\\\225 + 324=c^2\\\\549=c^2\\\\√(549)=c`

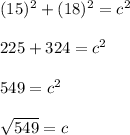
Use the Pythagorean theorem again in the right triangle with the legs (18) and (x)

`(x)^2+(18)^2=c^2\\\\√(x^2+324)=c`

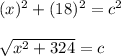
Use the Pythagorean theorem one final time in the larger triangle,

`(√(x^2+324))^2+(√(549))^2=(x+15)^2\\`

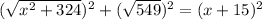
Simplify, remember that anything to the power (2) is equal to the base (number under the exponent) times itself,

`x^2+324+549=x^2+30x+225`

`x^2+873=x^2+30x+225`

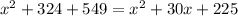
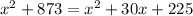
Inverse operations,

`x^2+873=x^2+30x+225\\-x^2\\\\873=30x+225\\-225\\\\648=30x\\/30\\\\(648)/(30)=x\\\\21.6=x`