Question

Foresters want to estimate the average age of trees in a stand. Determining age is cumbersome, because one needs to count the tree rings on a core taken from the tree. In general, though, the older the tree, the larger the diameter, and diameter is easy to measure. The foresters measure the diameter of all 1132 trees and find that the population mean equals 10.3. They then randomly select 20 trees for age measurement

Answer 1

hello your question has some missing information attached below is the missing information

answer : mean ratio of estimate = 117.62

standard error ( SE ) = 3.9765

Explanation:

Given data : n = 20, μx = 10.3 , N = 1132

∑ age ( yi) = ( 125 + 119 + --------- + 99 ) = 2148

∑ ( Y - rX)^2 = 6116.728

mean of age ( y ) = ∑ y / 20 = 2148 / 20 = 107.4

mean of diameter ( x ) = ∑x /20 = 188.1 / 20 = 9.405

∴ ratio estimate = y /x = 107.4 / 9.41 = 11.4195

i) The mean of ratio estimate

= 11.4195 * μx = 11.4195 * 10.3 = 117.6204

ii) determine the standard error ( SE )

calculate ;
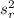`s^(2) _(r)` = 1 / ( n-1 ) * ∑ (Y - rX)^2 = 1/19 ( 6116.728 ) = 321.933

Var ( μr ) = [ ( N - n ) / N*n ]*
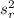`s^(2) _(r)` = [ ( 1132 - 20 ) / (1132*20) ] * 321.933

= 15.81226

therefore the SE = √15.81226 ≈ 3.9765