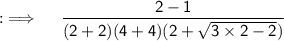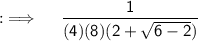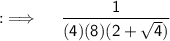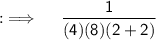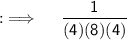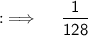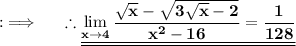We are given with a limit and we need to find it's value so let's start !!!!
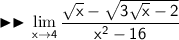
But , before starting , let's recall an identity which is the main key to answer this question
Consider The limit ;
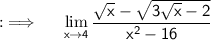
Now as directly putting the limit will lead to indeterminate form 0/0. So , Rationalizing the numerator i.e multiplying both numerator and denominator by the conjugate of numerator
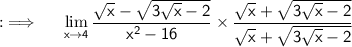
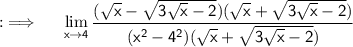
Using the above algebraic identity ;

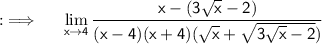
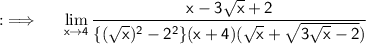
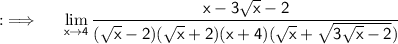
Now , here we need to eliminate (√x-2) from the denominator somehow , or the limit will again be indeterminate ,so if you think carefully as I thought after seeing the question i.e what if we add 4 and subtract 4 in numerator ? So let's try !
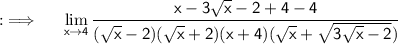
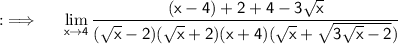
Now , using the same above identity ;
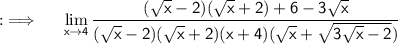
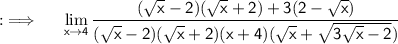
Now , take minus sign common in numerator from 2nd term , so that we can take (√x-2) common from both terms
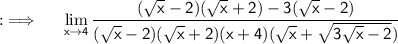
Now , take (√x-2) common in numerator ;
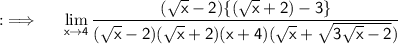
Cancelling the radical that makes our limit again and again indeterminate ;
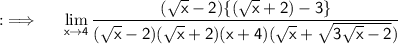
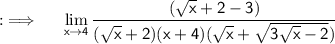
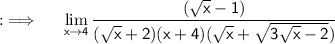
Now , putting the limit ;