Question

Can someone help me find the area

Answer 1

Area of the composite figure = 84 in²

Explanation:

Area of the composite figure = Area of rectangle DEFG + Area of rectangle BCGH + Area of triangle ABH

Area of rectangle DEFG = DE × EF

= 5 × 10

= 50 in²

From ΔBHA,

AB = ED = 5 in [Given]

AH = FA - (GH + FG)

= 15 - (7 + 5)

= 15 - 12

= 3 in.

By applying Pythagoras theorem in ΔABH,

AB² = HB² + AH²

5² = HB² + 3²

25 - 9 = HB²

HB = √16

HB = 4 in

Area of rectangle BCGH = BC × HB

= 7 × 4

= 28 square in.

Area of triangle ABH =
`(1)/(2)(\text{Height})(\text{Base})`

=
`(1)/(2)(BH)(AH)`

=
`(1)/(2)(4)(3)`

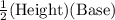
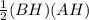
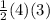
= 6 in²

Therefore, area of the composite figure = 50 + 28 + 6

= 84 in²