Question

A rectangle has a length of 17 less than 6 times it's width. If the area of the rectangle is 7585 square feet find the length of the rectangle​

Answer 1

9514 1404 393

Answer:

205 ft

Explanation:

Let w represent the width of the rectangle. Then the length is (6w-17) and the area is ...

A = LW

7585 = (6w-17)(w)

6w² -17w -7585 = 0

Perhaps most straightforward is using the quadratic formula to solve this.

For ax² +bx +c = 0, the solution is x = (-b±√(b²-4ac))/(2a). For the above quadratic, the solution is ...

`x=(-(-17)\pm√((-17)^2-4\cdot6\cdot(-7585)))/(2\cdot6)=(17\pm√(182329))/(12)\\\\x=(17\pm427)/(12)=\{-34(1)/(6),37\}`

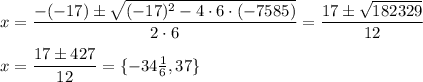
The width is 37 feet, so the length is ...

6(37) -17 = 205 . . . feet

The length of the rectangle is 205 feet.