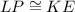Answer:
See Below.
Explanation:
We are given that:
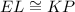
And we want to prove that:
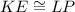
Congruent chords have congruent arcs. Therefore:
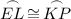
Arc EL is the sum of Arcs LP and PE:

Likewise, Arc KP is the sum of Arcs KE and PE:

Since Arcs EL and KP are congruent:
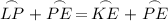
Subtraction Property of Equality:
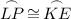
Congruent arcs have congruent chords. Therefore: