Question

The absorption of infrared (IR) radiation results in vibrations in the molecules or ions that make up a chemical sample. Vibrations cause bond lengths or angles to expand and contract. The energy of infrared radiation (i.e., frequency) required for a vibration depends on the type of bond and the mass of the atoms involved. Typically, the higher the bond order, the stronger the bond, and therefore, the more energy (i.e., higher frequency) required to make the bond vibrate. Also, lighter atoms vibrate at higher frequencies than heavier atoms. The order of vibrational frequencies (from largest to smallest) of the given carbon-nitrogen and carbon-sulfur bonds is

Answer 1

the order of vibrational frequency is:

C ≡ N > C=N > C=S > S-C

Step-by-step explanation:

The bond's vibration frequency is determined using the following equation:

`\zeta = (1)/(2 \pi c)\sqrt{(k)/(\mu)}`

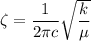
where;

reduced mass
`\mu = (m_1m_2)/(m_1+m_2)`

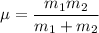
velcoity of light = c

force constant = k

The frequency of vibration, on the other hand, is inversely proportional to the atom's mass, because the heavier the atom, the lower the frequency.

In addition, the value of a bond's stretching frequency rises as the bond's intensity rises. As a result, the frequency is as follows:

triple > double > single

The reduced mass
`(\mu)` of C-N bond.

`\mu = (m_1m_2)/(m_1+m_2)`

`\mu = (12*14)/(12+14) \\ \\ \mu = 6.46`

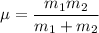
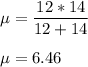
The reduced mass of C-S bond;

`\mu = (m_1m_2)/(m_1+m_2)`

`\mu = (12* 32)/(12+32) \\ \\ \mu = 8.72`

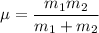
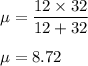
Thus, the order of vibrational frequency is:

C ≡ N > C=N > C=S > S-C