Answer:
the ratio of the etched to the original crack tip radius is 30.24
Step-by-step explanation:
Given the data in the question;
we determine the initial fracture stress using the following expression;
(σf)₁ = 2(σ₀)₁
 α₁/(
α₁/(
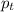 )₁
)₁
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png) ----- let this be equation 1
----- let this be equation 1
where; (σ₀)₁ is the initial fracture strength
(
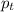 )₁ is the original crack tip radius
)₁ is the original crack tip radius
α₁ is the original crack length.
first, we determine the final crack length;
α₂ = α₁ - 16% of α₁
α₂ = α₁ - ( 0.16 × α₁)
α₂ = α₁ - 0.16α₁
α₂ = 0.84α₁
next, we calculate the final fracture stress;
the fracture strength is increased by a factor of 6;
(σ₀)₂ = 6( σ₀ )₁
Now, expression for the final fracture stress
(σf)₂ = 2(σ₀)₂
 α₂/(
α₂/(
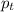 )₂
)₂
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png) ------- let this be equation 2
------- let this be equation 2
where (
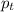 )₂ is the etched crack tip radius
)₂ is the etched crack tip radius
value of fracture stress of glass is constant
Now, we substitute 2(σ₀)₁
 α₁/(
α₁/(
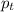 )₁
)₁
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png) from equation for (σf)₂ in equation 2.
from equation for (σf)₂ in equation 2.
0.84α₁ for α₂.
6( σ₀ )₁ for (σ₀)₂.
∴
2(σ₀)₁
 α₁/(
α₁/(
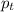 )₁
)₁
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png) = 2(6( σ₀ )₁)
= 2(6( σ₀ )₁)
 0.84α₁/(
0.84α₁/(
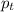 )₂
)₂
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png)
divide both sides by 2(σ₀)₁
 α₁/(
α₁/(
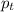 )₁
)₁
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png) = 6
= 6
 0.84α₁/(
0.84α₁/(
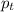 )₂
)₂
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png)
 1/(
1/(
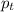 )₁
)₁
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png) = 6
= 6
 0.84/(
0.84/(
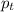 )₂
)₂
![]^{1/2](https://img.qammunity.org/2022/formulas/engineering/college/rvkq63c06skw5dcocmupqhvm7guz3zh82e.png)
 1/(
1/(
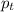 )₁
)₁
![]](https://img.qammunity.org/2022/formulas/mathematics/middle-school/6k6epemxei0igaktrm2pw36fy23c4xckrh.png) = 36
= 36
 0.84/(
0.84/(
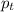 )₂
)₂
![]](https://img.qammunity.org/2022/formulas/mathematics/middle-school/6k6epemxei0igaktrm2pw36fy23c4xckrh.png)
1 / (
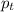 )₁ = 30.24 / (
)₁ = 30.24 / (
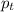 )₂
)₂
(
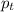 )₂ = 30.24(
)₂ = 30.24(
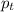 )₁
)₁
(
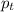 )₂/(
)₂/(
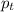 )₁ = 30.24
)₁ = 30.24
Therefore, the ratio of the etched to the original crack tip radius is 30.24