Answer:
a) The total engine volume is 30.3265 in³
b) the mass of air contained in the engine is 0.00129 lbm
c) The efficiency of the engine is 55.6%
Explanation:
Given the data in the question;
The configuration is in line with four-stroke, four-cylinder engine.
Bore ( d ) = 3.17 in
Stroke ( L ) = 3.48 in
compression ratio ( r ) = 10.6 : 1
First, we calculate the swept Volume V₁ = π/4 × d²L
we substitute
V₁ = π/4 × (3.17 )² × (3.48) = 27.4655 in³
Now, Compression Ration; r = Vs + Vc / Vc
r = (V₁ + V₂) / V₂
we substitute
10.6 = (27.4655 + V₂) / V₂
10.6V₂ = 27.4655 + V₂
10.6V₂ - V₂ = 27.4655
9.6V₂ = 27.4655
V₂ = 2.861 in³
So
a) total engine volume
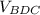 = V₁ + V₂
= V₁ + V₂
we substitute
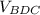 = 27.4655 in³ + 2.861 in³
= 27.4655 in³ + 2.861 in³
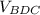 = 27.4655 in³ + 2.861 in³
= 27.4655 in³ + 2.861 in³
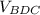 = 30.3265 in³
= 30.3265 in³
Therefore, The total engine volume is 30.3265 in³
b) The mass of air
given that; T = 540°R, P = 14.7 psia
we know that; PV = mRT { R is constant }
we solve for m
m = PV / RT
we substitute
m = (14.7 × 30.3265) / ( 0.3704 × 1728 )540
m = 445.79955 / 345627.648
m = 0.00129 lbm
Therefore, the mass of air contained in the engine is 0.00129 lbm
c) The efficiency
from table { properties of air}
At 540°R; u₁ = 92.04 BTu/lbm, u
 ₁ = 144.32
₁ = 144.32
Now process 1 - 2 ( Isentropic ) u
 ₁ / u
₁ / u
 ₂ = V₁/V₁ = r
₂ = V₁/V₁ = r
so, u
 ₁ / u
₁ / u
 ₂ = r
₂ = r
144.32 / u
 ₂ = 10.6
₂ = 10.6
u
 ₂ = 144.32/10.6
₂ = 144.32/10.6
u
 ₂ = 13.61
₂ = 13.61
Thus, at u
 ₂ = 13.61, u₂ = 235 BTU/lbm, T₂ = 1340°R
₂ = 13.61, u₂ = 235 BTU/lbm, T₂ = 1340°R
Now, we know that;
P₁V₁/T₁ = P₂V₂/T₂ ⇒ (P₁/V₁)r = P₂/T₂
⇒ ( 14.7 / 540 )10.6 = P₂/1340
P₂ = 386.664 psia
process 2 - 3; constant volume heat addition;
Now, At T₃ = 3600°R, u₃ = 721.44 BTU/lbm, u
 ₃ = 0.6449
₃ = 0.6449
so,
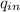 = u₃ - u₂
= u₃ - u₂
we substitute
so,
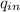 = 721.44 - 235 = 486.44 BTU/lbm
= 721.44 - 235 = 486.44 BTU/lbm
Next, process 3 - 4; Isentropic
u
 ₄/u
₄/u
 ₃ = V₄/V₃ = r
₃ = V₄/V₃ = r
u
 ₄ / 0.6449 = 10.6
₄ / 0.6449 = 10.6
u
 ₄ = 6.8359
₄ = 6.8359
At u
 ₄ = 6.8359; u₄ = 308 BTU/lbm
₄ = 6.8359; u₄ = 308 BTU/lbm
∴
Heat Rejection
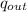 = u₄ - u₁ = 308 - 92.04 = 215.96 BTU/lbm
= u₄ - u₁ = 308 - 92.04 = 215.96 BTU/lbm
so,
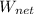 =
=
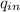 -
-
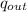
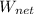 = 486.44 - 215.96
= 486.44 - 215.96
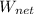 = 270.48 BTU/lbm
= 270.48 BTU/lbm
so the efficiency; η
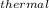 = (
= (
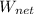 /
/
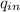 ) × 100%
) × 100%
η
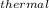 = ( 270.48 / 486.44 ) × 100%
= ( 270.48 / 486.44 ) × 100%
η
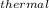 = ( 0.55604 ) × 100%
= ( 0.55604 ) × 100%
η
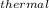 = 55.6%
= 55.6%
Therefore, The efficiency of the engine is 55.6%